Tag: Probability

The Birthday Paradox

By Anupum Pant

Imagine you meet a random person in the street and ask him/her when is their birthday, there’s a huge chance that the person’s birthday will not be the same as your birthday. In fact, the probability of both your birthdays being on the same day is around 0.27%. Fat chance. At the back of our heads, this is something that is very clear to all of us.

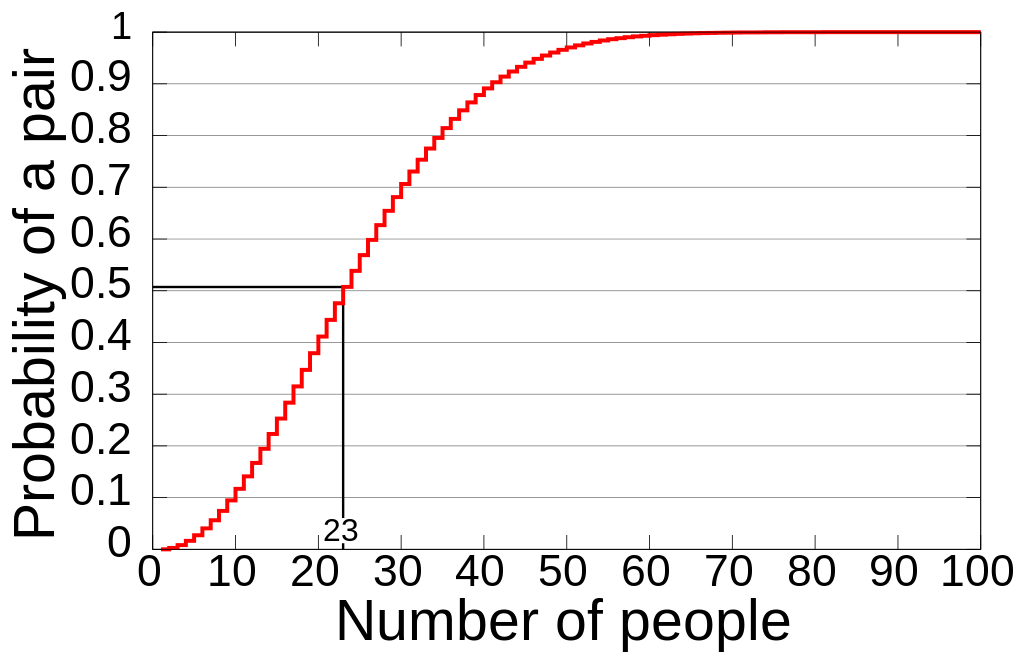
Again, if you repeat this by asking about 22 people the same question, the chance of you finding someone having the same birthday as yours is still around 5%. Too less. This is too is a very intuitive piece of information.

But consider this. If I put all of the 22 guys and you in a room, there’s a big chance that 2 people in that room will have the same birthday – a 50% chance. Moreover, if there are 70 people in the room, this chance increases to about 99.99%. This is called the birthday problem or the birthday paradox.

So, what changed when 20 people went into the room? It was just the fact that in the room, we are picking 2 people from a group of 23 people. That is equivalent to this – everyone is asking everyone their birth dates. Everyone doing it simultaneously makes the probability much higher. The probability of two people sharing a birth date among a group of 23 people is far higher than you alone going around and asking all the 22 people, and finding someone having the same birthday as your’s.

Suppose there are 200 people in the room. The probability of 2 people sharing their birthday is massive (and yet not definite). There is in fact a 99.9999999999999999999999999998% chance!

1024px-Birthday_Paradox.svg

Finally, if you had 367 people in a room, at least a pair among these 367 people in the room would definitely have the same birth date. The 99.99% chance shoots up to a definite (100%) probability if there are 367 people in the same room. Think about it for a minute.

Using Psychology To Get Back Your Lost Wallet

By Anupum Pant

Do you like to keep a picture of someone you love in your wallet? If the answer is no, you should probably start doing it. But, suppose you have a loved baby, adorable parents, cute puppy and grandparents at home, all of whom you love equally, whose picture do you think would be the best one to keep in your wallet?

Professor Richard Wiseman from University of Hertfordshire, a psychologist, decided to find out. He designed an experiment that would be conducted on the street and would help him figure out the answer to this tough choice.

An experiment on the street

He and his team dropped 240 wallets around the city of Edinburgh. Just to find out, how many of the wallets would be returned by the finders to their respective owners.

Not all the wallets were same. A few displayed picture of a cute baby, others had a picture of a puppy, some had a family picture and others contained an elderly couple’s portrait.

There were some other wallets dropped which contained a receipt suggesting how charitable the owner of that wallet was. These had no pictures in them.

Which one do you think won? Guess and read on…

Results!

Following were the return percentages of wallets:

  • I hope babies don’t get too much cute-aggression out of you because the ones with baby pictures – An incredible 88% of these wallets got returned!
  • Ones with the puppy pictures – 53% were returned.
  • Family portrait wallets – 48% came back.
  • With just 28% return percentage, the ones with the picture of an elderly couple fared the worst among all wallets that had pictures.
  • And only 15% of the wallets that enclosed a receipt and had no pictures were returned to their owners.

Moral (take it with a grain of salt)

If it doesn’t hurt, you could experiment with a cute baby’s picture in your wallet. Since it was tested in just one city, there is a great chance that you could get a different result in your area. If you don’t have one yet, find one on the WWW. The internet is full of them!

Getting back a lost wallet 88 times out of 100 times is big probability. What do you have to lose? A simple picture of a baby will pump up your chances of getting back the wallet by so many percentage points. Go, get one printed right now!

Related articles
Enhanced by Zemanta

Gompertz Law – The Dreadful Law of Death

By Anupum Pant

There is no astrologer in the world that can tell you for sure if you’ll die this year or not. But, thanks to Gompertz Law, if you ask me, there is one thing I can tell you for sure – Whatever may be the odds of you dying this year, in 8 years, the likelihood of you dying will double.

This dreadful law of death was named after the first person who noted it – Benjamin Gompertz, in the year 1825. The law rests on a general assumption that a person’s resistance to death decreases as he ages. The Gompertz Law of mortality, put simply in a sentence would compute to this:

Your probability of dying during a given year doubles every 8 years.

It is amazing, and no one knows how it works exactly. Why does nature pick the number 8, to double our likelihood of death? We’ll probably never know.

There is a whole table which relies on census data, and statistically notes the probabilities of people dying at different ages. And when it is plotted on a Probability of death vs. Age graph, you get an exponentially increasing mortality rate with age. That is death coming faster as you get older.

Gompertz Law can be verified for real-life data – the 2005 US census data. The following graph and the probability vs. age plotted using the law match almost perfectly. Amazingly, the law holds true for several other countries too.

gompertz law graph

That means, the probability of me, a 25-year-old dying during the next year is very small — about 1 in 3,000. When I become 33, this probability will grow to something around 1 in 1,500. In the next 8 years, the probability of me dying will be 1 in 750, and so on…At the age 100, the probability a person’s death will be about 1 out of 2 – fat chance of successfully moving on to 101!

Theoretically, using this data, it can be said with 99.999999% certainty that no human will ever live to the age of 130 (of course only if medicine doesn’t start tampering with human genes, or some other artificial factor). There is one thing for sure – there is almost no chance that you are going to beat Mr. Ming.

If you liked this article, do subscribe to my daily newsletter to receive the full articles in your mail everyday. What could be better way to make sure that you learn at least one new thing everyday.