By Anupum Pant
Abraham Lincoln, the 16th president of the United States, was probably a math whiz. Doesn’t it sound like an extremely rare combination of things a person could possibly be? – a president and a math whiz. And yet, he was not the only one. James Garfield, the 20th president, was very much into mathematics too.
Garfield wasn’t a professional mathematician. He was a president. But, much like Abraham Lincoln, he was very much into geometry! Before he went into politics, he wanted to become a mathematics professor.
While he was a member of the US house of representatives, five years before he was elected president of the US, he came out with a very elegant and unique proof of the Pythagorean theorem (yes, another one of those Pythagorean theorem proofs). Here’s how he did it with the help of a congruent flipped triangle…
Doing it with a piece of paper is really easy…
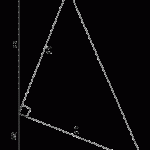 Fold a paper and cut 2 exactly same right-angled triangles out of it. Now, put them together as shown in the image here (click the image). Next, write down the area of the trapezium – (a + b) . ½(a + b) – 1
Fold a paper and cut 2 exactly same right-angled triangles out of it. Now, put them together as shown in the image here (click the image). Next, write down the area of the trapezium – (a + b) . ½(a + b) – 1
Now write the area of all the 3 triangles and add them. This is what you’d get – 2 x ½ ab + ½ c2 – 2
Since both these areas are same, just written in a different way, equate them and solve. You’ll end up with the Pythagorean theorem!
a2 + b2 = c2
Or, simply watch the video to understand better…