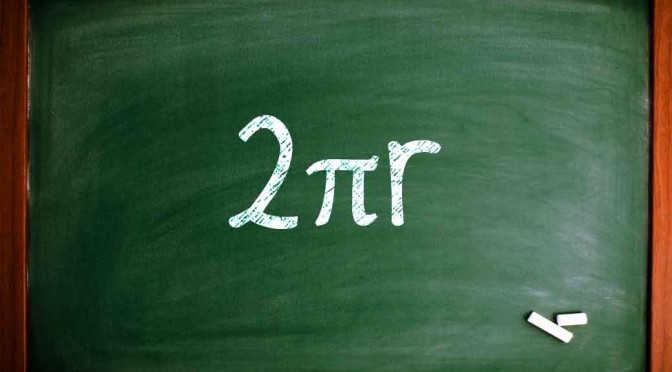Tag: Golf ball

A Thread Around the Earth

By Anupum Pant

Background

Couple of days back, I read about a puzzling geometrical conundrum, probably on Quora. It might not sound amusing to you math geeks out there, but to me, it sounded like an impossible thing at first. The sad part is, I did not save the source link in my notes. Thankfully, I did care to note down the idea. Let me call it the “Thread wound Around the Earth” puzzle.

Here is a simple question first. Try answering it without any calculation. Just guess. Be honest to yourself, don’t see the answer just below it. Scroll slow.

As BBC’s website puts it…

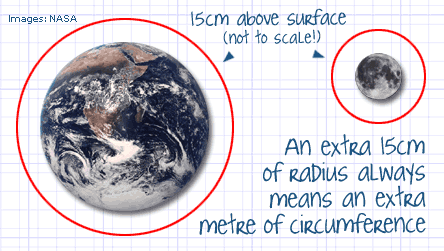
Imagine a piece of string wrapped around the Earth’s equator – that’s about 40,000km. How much MORE string would you need for it to sit 15cm above the ground, all the way around?

A) 1 metre, B) 1 kilometre or C) 1,000 kilometres

Thread wound around the Earth

The answer is A) 1 meter. Yes, just 1 meter of extra rope.

Suppose, you have an outrageously long thread with you. You tie it around the base of a tree, somewhere at the equator. Now, you go around the earth, along the equator, carrying the thread with you, till you come back to that tree where you started. At this point, you’ll have a thread that goes around the earth in a circle. At every point, let us imagine that the rope is taut and touching the ground (there are no mountains or valleys in between). It’s a perfect circle (assume).

Suppose, you still have an extra meter of the rope left now. So, you break the wound rope at one point and add the extra meter to it. That of course slackens the wound rope. For this rope to be taut again, it has to be lifted up by some amount. What do you think that distance would be from the ground? Assume that the rope still makes a huge circle just above the ground and lifts by equal amount at every point along the equator.

Just the extra meter of rope, causes the rope to rise by ~15 cm all around the earth (actually 15.9 cm). For a single meter of rope added to a 40,000 km of rope, that sure seems like a huge lift! But that isn’t all…

rope 15 cm above earth

The most amazing part is that, no matter what the size of the circle, a meter of increased circumference will increase the radius by ~15 cm. Always!

Try tying a rope around a golf ball, or even try doing that around the sun. It’s always that – 1 meter increase in circumference, always increases the radius by ~15 cm.

The Math is so straightforward.

If you think about it mathematically, it is completely straightforward.

Radius X 2 X Pi = Circumference

That means, the Radius is directly proportional to the circumference of a circle. Everyone knows that. So, the amount of change in the radius is reflected proportionally in the circumference, the magnitude of radius can be anything, really. So it’s pretty natural that just a single meter of rope is required to lift the rope by 15.9 cm around any circle. The size doesn’t matter. But practically thinking, the above question makes it seem impossible.

Please hit like if you learnt something from the article.

Meet a 12-year-old Scientist – Peyton Robertson

By Anupum Pant

Today we meet a 12-year-old ‘man’ who has been on an invention spree since he was just 8 years old. If I may use a pop-culture reference, this adorable boy is a Sheldon Copper in the making.

Peyton Robertson from Fort Lauderdale, Florida, presently has 3 patents pending:

  1. A case (box) to maintain a resting golf ball’s temperature – Peyton loves golf. And on one cold day, when he observed that his golf balls weren’t bouncing the way they should have been, he, instead of sleeping on the problem, thought of finding a solution.
  2. Retractable training wheels: This one is a pair of retractable training wheels connected to a lever mechanism on the handle of a bicycle – you press the lever and the training wheels rise up. A perfect solution for kids who want to experience the joy of biking while they are learning to ride. He invented this to help his sister when she was learning to ride a bicycle. Today, bike manufacturers are flocking around him to buy his idea.
  3. A sand-less sand bag: When the super-storm Sandy struck 24 US states in October last year, the entire eastern sea-face from Florida to Maine suffered great losses. It caused a damage of around $65 billion. Peyton saw this and figured that the sand bags that were being used for flood defense contributed to a lot of inefficiency. These bags were 40 pounds each; moving them from one place to another was tough. But they had to be heavy to stop the water. Besides that, the bags when stacked left undesirable gaps in between, which caused a leakage. Peyton felt a need to contribute to make people better equipped for floods in the future.

His solution – A sand-less sand bag – is better than the traditional bag in two ways. Firstly, weighing around 4 pounds, it is significantly lighter than the sand bag. It contains a mixture of, a polymer that expands when it comes in contact with water, and salt which makes it heavy when it wets. Secondly, this bag comes with an interlocking fastener which keeps the bag in place when it expands – Removing any gaps which could create a leak during the floods. Moreover these bags can be dried later to be reused.

The witty sandbag made him the youngest ever person to win the Discovery Young Scientist Challenge. The prize – $25,000 and a trip to Costa Rica!

In an interview with TED blog he said:

Failure is progress and a normal part of the process. Whether it’s science or life, you have to start, fail and just keep pushing. In a football game, time runs out, and a golf match ends after the last hole. But when you are working on something and it doesn’t work, you just extend the game – and give your experiment or your prototype another go.

It was a delight to watch the charming boy speak on The Ellen DeGeneres Show. Sadly, that video no longer exists, here’s a replacement with the guy’s own pitch: